Class 11 CBSE Applied Maths Mensuration Exercise 4.2
Class 11 CBSE Applied Maths aims to develop an understanding of basic
mathematical and statistical tools and their
applications in the field of commerce (business/ finance/economics) and social
sciences. Topics covered in Class 11th Applied Maths includes : Numbers, Quantification and
Numerical Applications, Algebra, Calculus, Probability Distributions , Inferential Statistics, Index
Numbers and Time-based data , Financial Mathematics , Linear Programming.
Please Select
Q1. Dimensions of the cuboidal hall are 10 m x 12 m x 15 m. Find the length of the longest rod that can be fitted into the hall.
Solution :

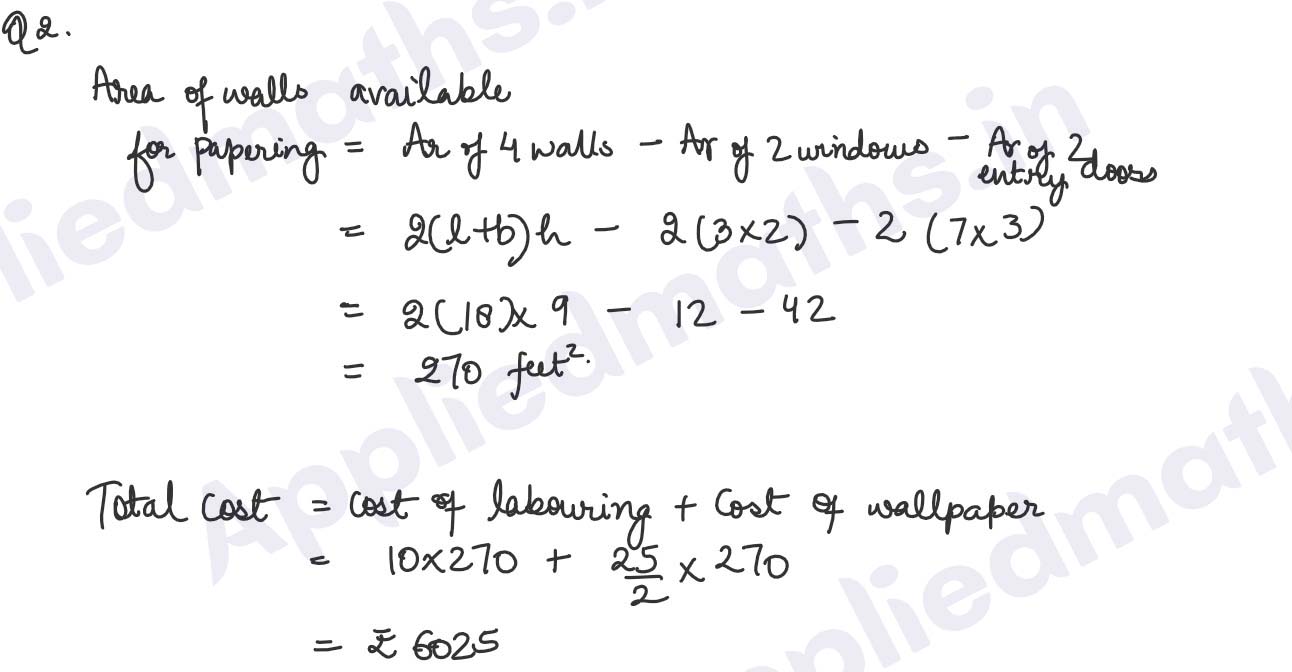
Q2. Nishant owns a house of dimensions 10 feet x 8 feet x 9 feet. His house has two entry doors on the opposite sides, of dimensions 7 feet by 3 feet each and two windows on opposite walls of dimensions 3 feet x 2 feet each. He wants wall papering to be done in his house. If the wallpaper that he selects is 2 feet wide and the cost is Rs 25 per feet. Find the total cost paid by Nishant. If the labour charges Rs 10 per sq. feet.
Solution :
Q3. The volume of a cuboidal block of silver is 10368 cm3. If its dimensions are in the ratio 3:2:1, find (i) the dimensions of the block. (ii) the cost of gold polishing its entire surface at Rs 0.50 per cm2,
Solution :

Q4. A rectangular plot is 24 m long and 20 m wide. A cubical pit of edge 4 m is dug at each of the four comers of the field and the soil removed is evenly spread over the remaining part of the plot. By what height does the remaining plot get raised?
Solution :

Q5. A rectangular container whose base is a square of side 6 cm, stands on a horizontal table and holds water upto 1 cm from the top. When a cube is placed in the water and is completely submerged, the water rises to the top and 2 cm3 of water overflows. Calculate the volume of the cube.
Solution :
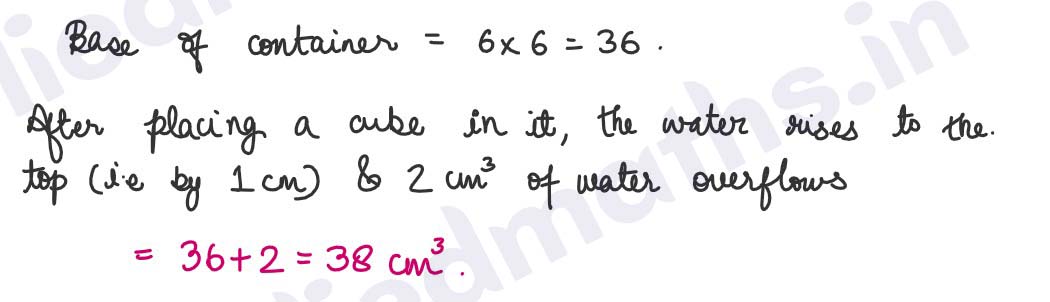
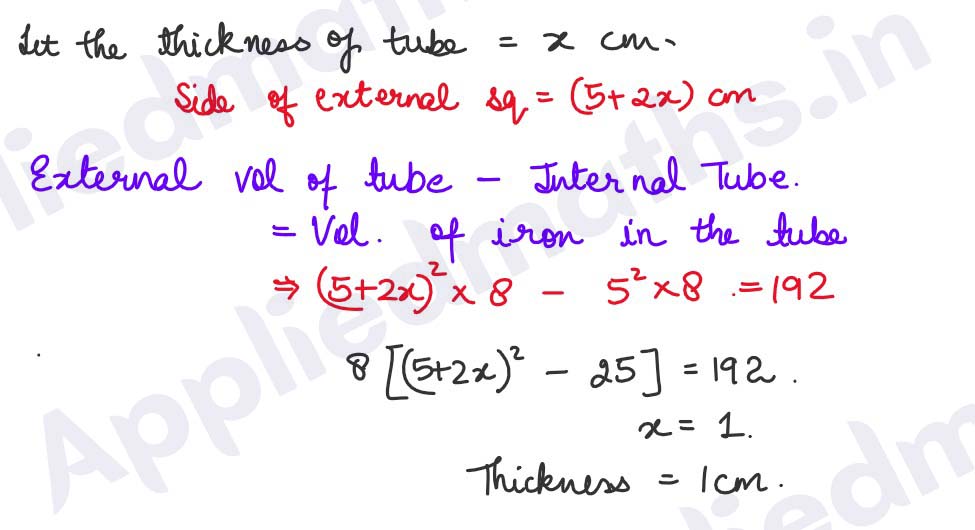
Q6. A hollow square shaped tube open at both ends, is made of iron. The internal square is of 5 cm side and the length of the tube is 8 cm. There are 192 cm3 of iron in this tube. Find its thickness.
Solution :
Q7. A rectangular pit 1.4 m long, 90 cm broad and 70 cm deep was dug and 1000 bricks of base 21 cm by 10.5 cm were made from the earth dug out. Find the height of each brick.
Solution :
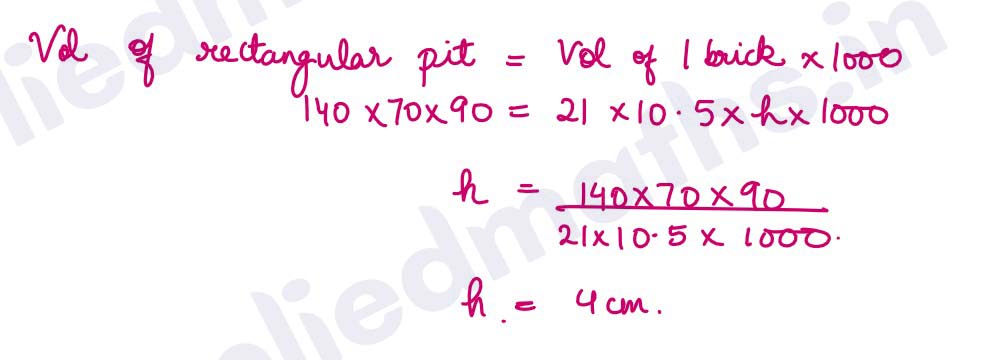
Q8. The inner dimensions of a closed wooden box are 2 m, 1.2 m and 0.75 m. The thickness of the wood is 2.5 cm. Find the cost of wood required to make the box if 1 m3 of wood costs Rs 5400.
Solution :

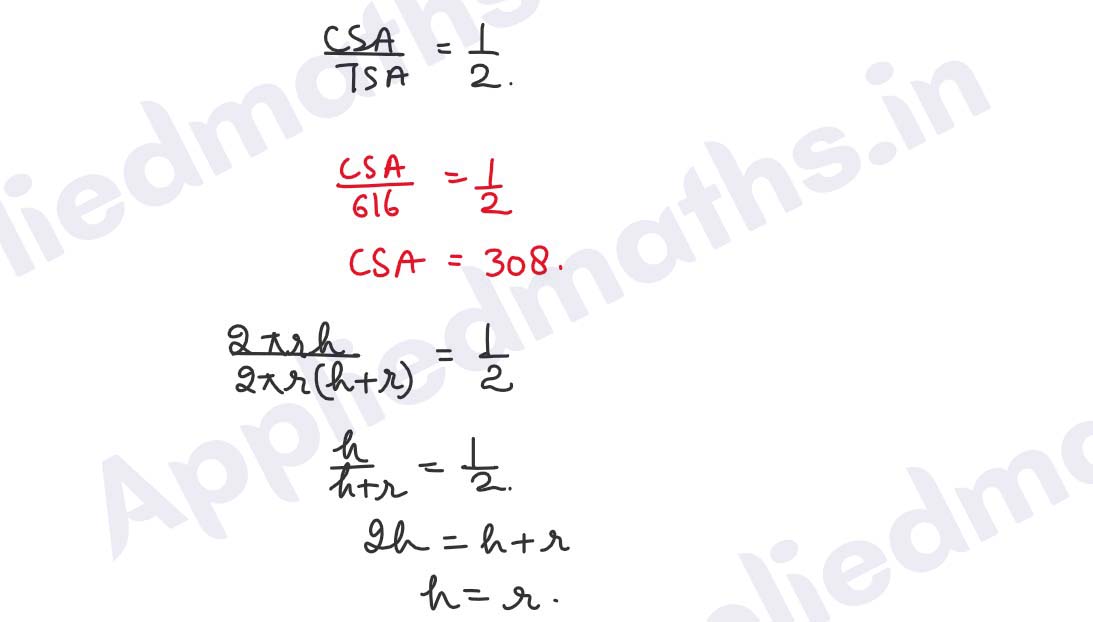
Q9. The ratio between the curved surface area and the total surface area of a right circular cylinder is 1 : 2. If the total surface area of the cylinder is 616 cm2, then find volume.
Solution :

Q10. Rainwater which falls on a flat rectangular surface of length 6 m and breadth 4 m is transferred into a cylindrical vessel of internal radius 20 cm. What will be the height of water in the cylindrical vessel if the rain fall is 1 cm? Give your answer to the nearest integer. (Take π = 3.14)
Solution :

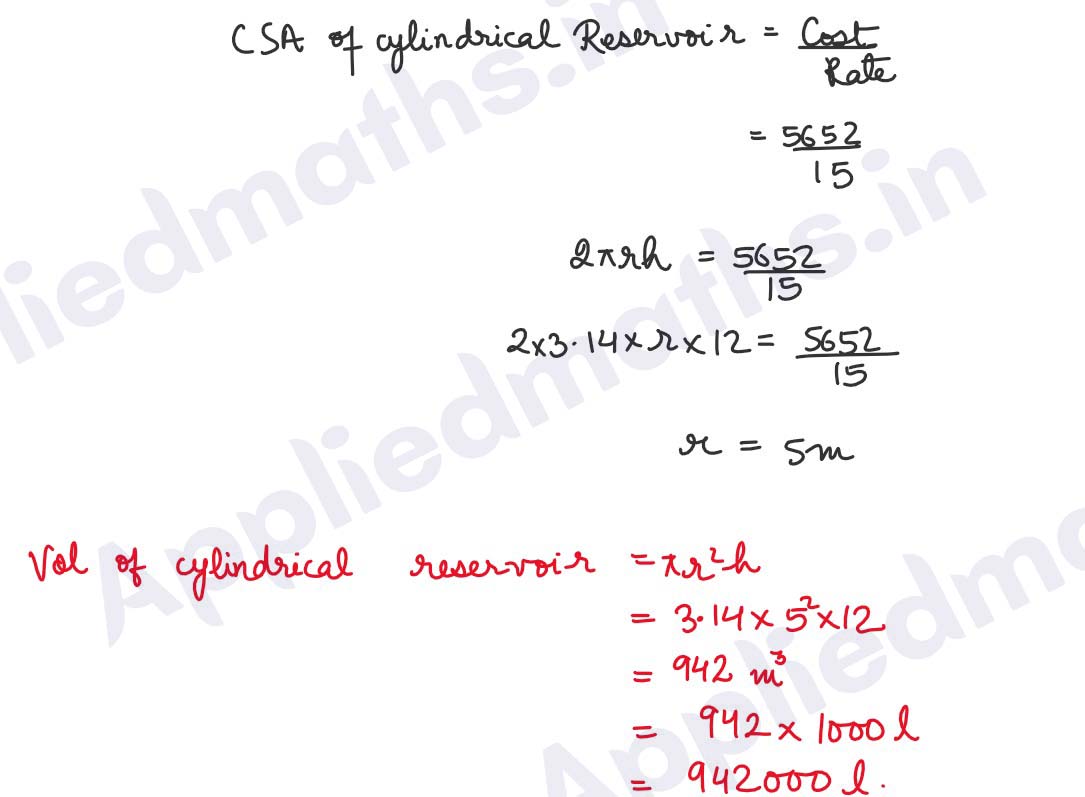
Q11. Curved surface of cylindrical reservoir 12 m deep is plastered from inside with concrete mixture at the rate of Rs 15 per m2. If the total payment made is Rs 5652, then find the capacity of this reservoir in litres. (Use π = 3.14).
Solution :
Q12. If the height and radius of a cone is tripled, then find the ratio of volume of new cone and that of the original cone.
Solution :

Q13.
Acone is surmounted on a hemisphere as shown in
adjoining figure. The radius of the solid formed is
6 cm and its height is 21 cm, find
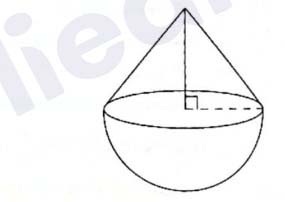
(i) curved surface area of the solid.
(ii) volume of the solid.
Solution :

Q14. A cuboidal gold block of dimensions 9 cm x 11 cm x 12 cm is melted and recasted into spherical balls of radius 3 mm. Find the number of spherical balls formed.
Solution :
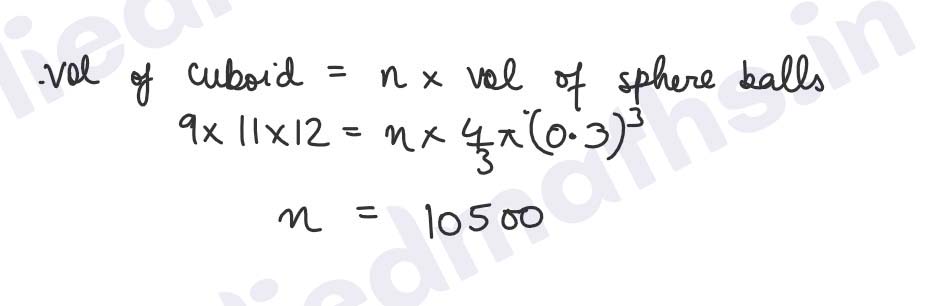
Q15. Monica has a piece of canvas whose area is 552 m2. She uses it to make a conical tent witha base radius of 7 m. Assuming that all the stitching margins and the wastage incurred while cutting, amounts to approximately 2 m2, find the volume of the tent that can be made with it.
Solution :
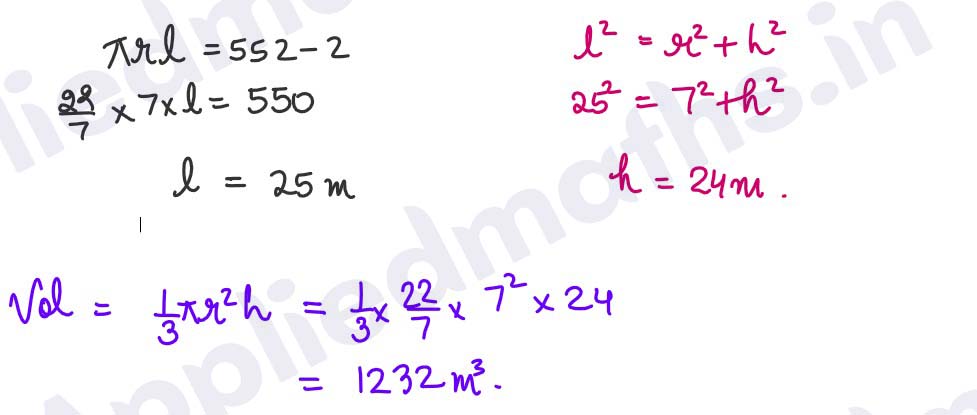
Q16.
The volume of a conical tent is 1232 m3 and the area of the base floor is 154 m2. Calculate
the:
(i) radius of the floor. (ii) height of the tent.
(iif) length of the canvas required to cover this conical tent if its width is 2 m.
Solution :

Q17. Find the radius and the curved surface area of the cone made from a quadrant of a circle of radius 28 cm.
Solution :

Q18. A hemispheral dome of a building needs to be painted from outside if the circumference of the base of the dome is 17.6m find the cost of painting it at the rate of Rs 8 per 100 cm2?
Solution :
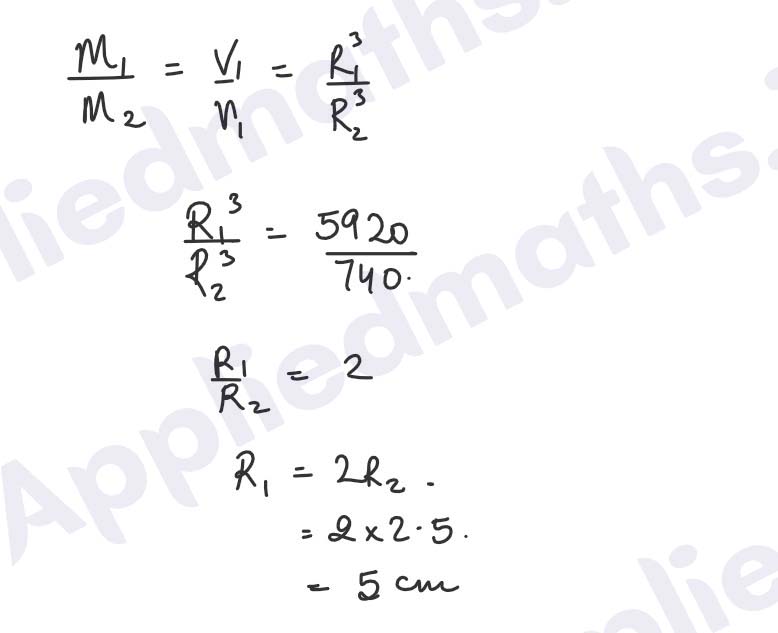
Q19. Two solid spares made of the same metal have weighs 5920 g and 740 g respectively determine the radius of the largest sphere if the diameter of the smaller one is 5cm
Solution :

Q20. The water for a factory is stored in a hemispherical tank whose internal diameter is 14m , the tank consist 50 kilolitres of water, water is pumped into the tank to fill to its capacity and the volume of the water pump into the tank.
Solution :
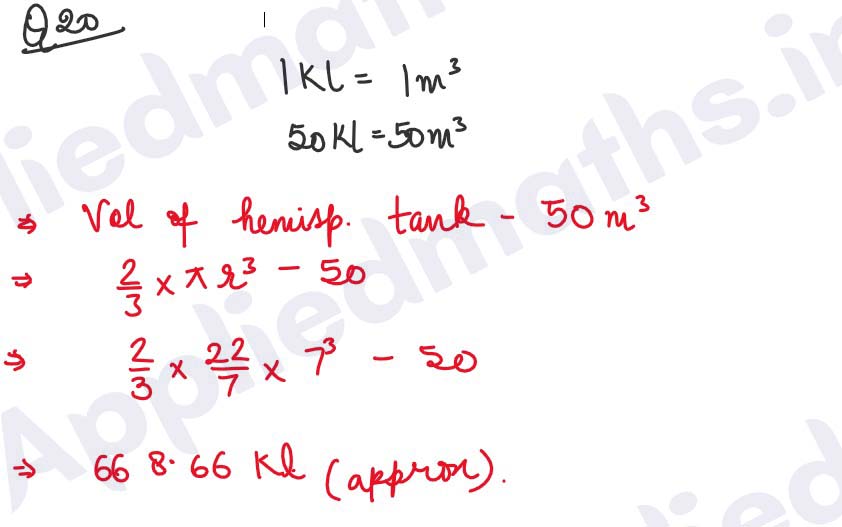
Q21. A cone of maximum size is carved out from a cube of edge 14 cm. Find the surface area of the cone and remaining solid left out after the cone carved out
Solution :

Q22.
The adjoining diagram shows a model of a rocket consisting of a cylinder surmounted end. by a de at a The dimensions of the model are : common radius 3.cm, height of cone = 4 cm and total height = 14 cm. If the model is drawn to a scale of 1:500, find
(i) the total surface area of the rocket in πm2.
(ii) the total volume of the rocket in m3.

Solution :

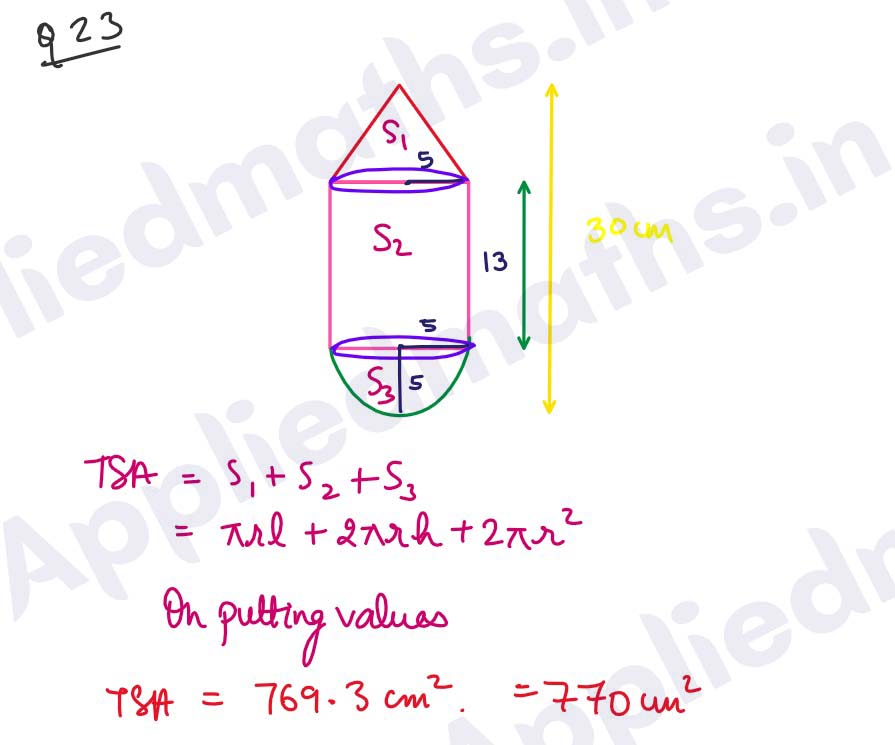
Q23. A toy is in the shape of a right circular cylinder with a hemisphere on one end and a cone on the other. The height and radius of the cylindrical part are 13 cm and 5 cm respectively. The radii of the hemispherical and conical parts are the same as that of the cylindrical part. Calculate the surface area of the toy if the height of the conical part is 12 cm.
Solution :
Q24. A girl fills a cylindrical bucket 32 cm in height and 18 cm in radius with sand. She empties the bucket on the ground and makes a conical heap of the sand. If the height of the conical heap is 24 cm, find (i) the radius and (ii) the slant height of the heap.
Solution :

Q25. A hollow sphere of internal and external radii 6 cm and 8 cm respectively is melted and recast. into small cones of base radius 2 cm and height 8 cm. Find the number of cones formed.
Solution :
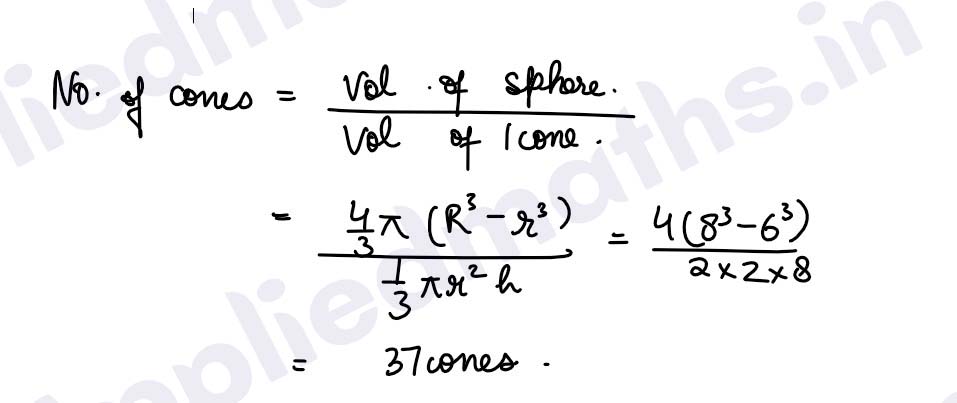
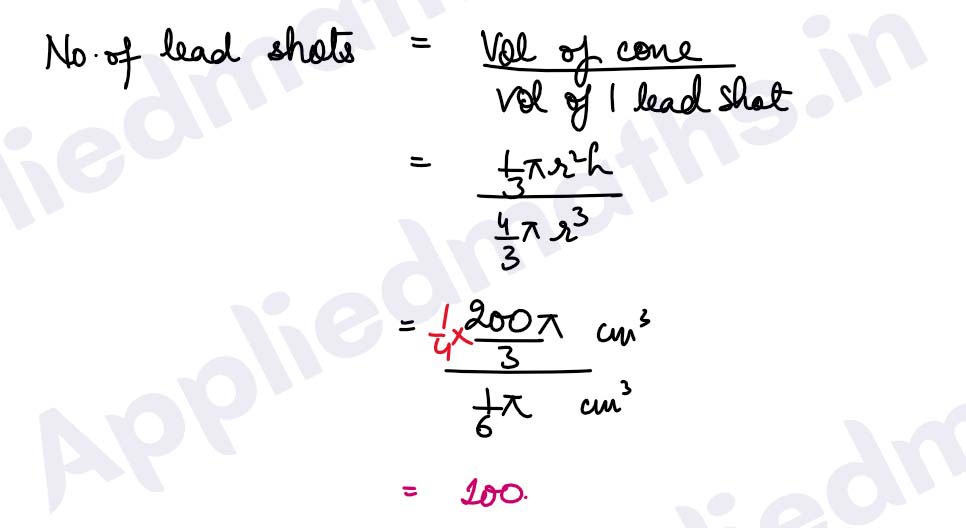
Q26. A vessel is in the form of an inverted cone. Its height is 8 cm and the radius of its top, which is open, is 5 cm. It is filled with water upto the brim. When lead shots, each of which is a sphere of radius 0.5 cm are dropped into the vessel, one-fourth of the water flows out. Find the number of lead shots dropped into the vessel.
Solution :
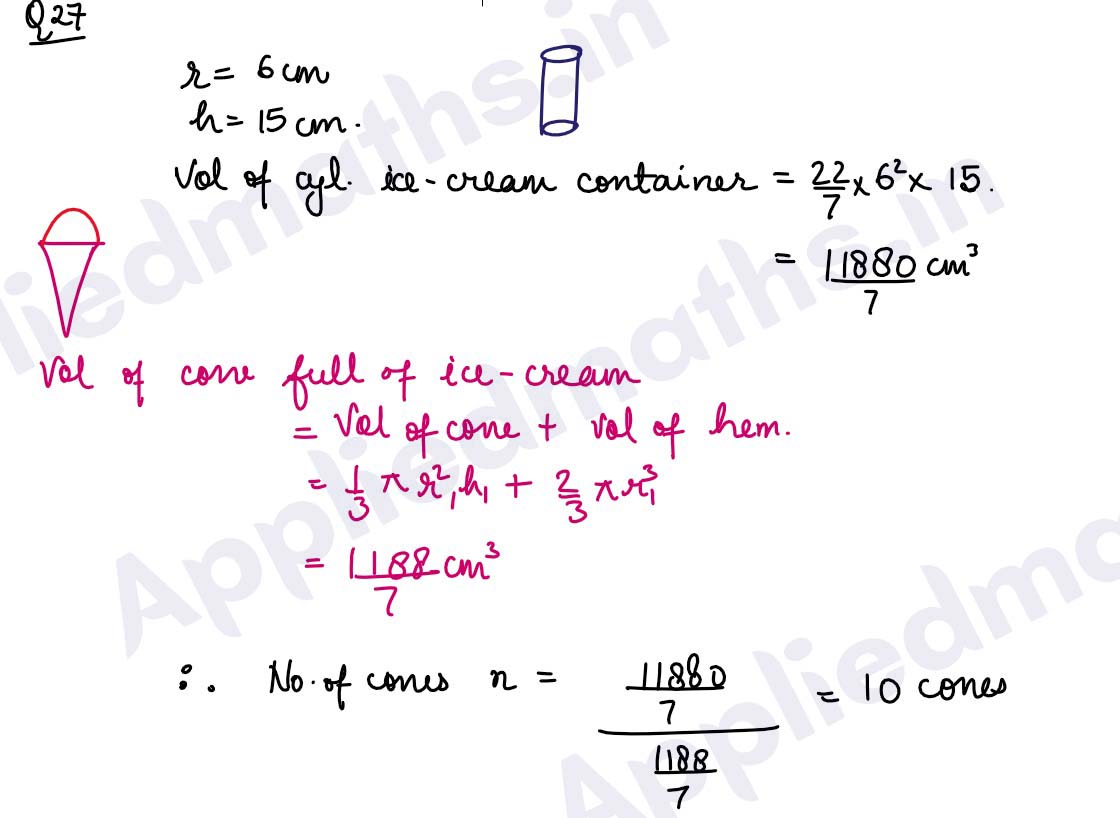
Q27. A container shaped like a right circular cylinder having diameter 12 cm and height 15 cm is full of icecream. The icecream is to be filled into cones of height 12 cm and diameter 6 cm, having a hemispherical shape on the top. Find the number of such cones which can be filled with icecream
Solution :